Quiz-summary
0 of 10 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
Information
Series Circuits Calculation Practice Test Questions Answers:
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Results
0 of 10 questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 points, (0)
| Average score |
|
| Your score |
|
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- Answered
- Review
-
Question 1 of 10
1. Question
1 pointsWhat will be the equivalent Resistance in the given Circuit:
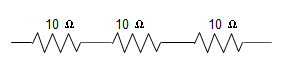 Correct
Correct
In the Circuit Three Resistances are connected in series.
In Series Circuit Req = R1 + R2 + . . .+ Rn
Req = 10 + 10 + 10 = 30ΩAnswer: 30Ω
Incorrect
In the Circuit Three Resistances are connected in series.
In Series Circuit Req = R1 + R2 + . . .+ Rn
Req = 10 + 10 + 10 = 30ΩAnswer: 30Ω
-
Question 2 of 10
2. Question
1 pointsCalculate the Current flowing through the Circuit:
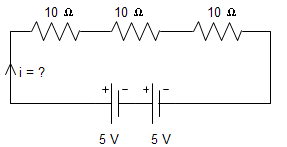 Correct
Correct
Voltage in the Circuit = 5 + 5 = 10V
Resistance in the Circuit = 10 + 10 + 10 = 30 ohms
Current in the Circuit = (V/I) = (10/30) = 0.334 AAnswer: i = 0.334A
Incorrect
Voltage in the Circuit = 5 + 5 = 10V
Resistance in the Circuit = 10 + 10 + 10 = 30 ohms
Current in the Circuit = (V/I) = (10/30) = 0.334 AAnswer: i = 0.334A
-
Question 3 of 10
3. Question
1 pointsWhat is the equivalent ICapacitance value of the Circuit:
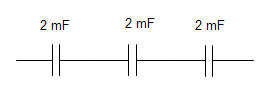
Correct
The Capacitances are connected in parallel in the Circuit:
1/Ceq = 1/C1 + 1/C2 + … + 1/Cn
1/Ceq = 1/(2) + 1/(2) + 1/(2)
1/Ceq = 3/2
Ceq = 2/3Answer: 2/3 mF
Incorrect
The Capacitances are connected in parallel in the Circuit:
1/Ceq = 1/C1 + 1/C2 + … + 1/Cn
1/Ceq = 1/(2) + 1/(2) + 1/(2)
1/Ceq = 3/2
Ceq = 2/3Answer: 2/3 mF
-
Question 4 of 10
4. Question
1 pointsCalculate the equivalent Inductance Value of the Circuit:
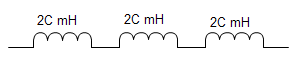 Correct
Correct
Inductors are Connected in Series
In a Series Circuit Leq = L1 + L2 + .. + Ln|
Leq = 2C + 2C + 2C = 6C mHAnswer: 6C mH
Incorrect
Inductors are Connected in Series
In a Series Circuit Leq = L1 + L2 + .. + Ln|
Leq = 2C + 2C + 2C = 6C mHAnswer: 6C mH
-
Question 5 of 10
5. Question
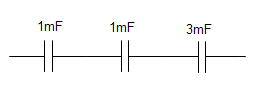
1 pointsCalculate the Equivalent Capacitance value:
 Correct
Correct
1/Ceq = 1/(1mF) + 1/(mF) + 1/(3 mF)
Ceq = 3/7 mFIncorrect
1/Ceq = 1/(1mF) + 1/(mF) + 1/(3 mF)
Ceq = 3/7 mF -
Question 6 of 10
6. Question
1 pointsCalculate the Voltage V in the given circuit:
 Correct
Correct
Current flowing through the Circuit = 5 A
Voltage across 5Ω Resistor = I x R = 5A x 5Ω = 25Answer: 25V
Incorrect
Current flowing through the Circuit = 5 A
Voltage across 5Ω Resistor = I x R = 5A x 5Ω = 25Answer: 25V
-
Question 7 of 10
7. Question
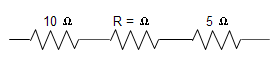
1 pointsIf the equivalent Resistance Value of the Circuit = 30Ω. What will the value of the unknown resistance:
 Correct
Correct
Equivalent Resistance Value = 30Ω
Resistance of the Circuit = 10 + 5 + R which is equal to 30Ω
Therefore 15 + R = 30; R = 15ΩAnswer: 15Ω
Incorrect
Equivalent Resistance Value = 30Ω
Resistance of the Circuit = 10 + 5 + R which is equal to 30Ω
Therefore 15 + R = 30; R = 15ΩAnswer: 15Ω
-
Question 8 of 10
8. Question
1 pointsFind the Value of Voltage V in the Circuit:
 Correct
Correct
Supply Voltage in the Circuit = 50 V
Current flowing through the Circuit = 5 A
Equivalent Resistance of the Circuit = (V)/(I) = (50/5) = 10 Ω
Equivalent Resistance of the Circuit given = 2 + 2 + R = 10; R = 6 ΩVoltage across 6Ω resistor = I x R = 6 x 5 = 30 VAnswer: 30 V
Incorrect
Supply Voltage in the Circuit = 50 V
Current flowing through the Circuit = 5 A
Equivalent Resistance of the Circuit = (V)/(I) = (50/5) = 10 Ω
Equivalent Resistance of the Circuit given = 2 + 2 + R = 10; R = 6 ΩVoltage across 6Ω resistor = I x R = 6 x 5 = 30 VAnswer: 30 V
-
Question 9 of 10
9. Question
1 pointsTwo Voltage sources V 1 and V 2 are connected in series as shown in the figure and equivalent voltage of the circuit is also given. What will be the condition:.
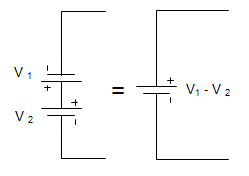 Correct
Correct
Incorrect
-
Question 10 of 10
10. Question
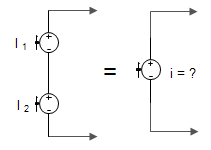
1 pointsTwo Current Sources are Connected in series, what is the condition to connect the circuit:
 Correct
Correct
Incorrect